AN-938: Digital and Analog Measurement Units for Digital CMOS Microphone Preamplifier ASICs
Introduction
The purpose of this application note is to explain the difference between analog and digital values when measuring the input and output of a digital microphone preamplifier with an audio analyzer. Specifically, the difference between peak and rms values, when measured digitally (FFS or dBFS) or in the analog domain (V or dBV), is examined. Some sample calculations in the following sections illustrate the necessary conversions between the various definitions.
Units in Digital and Analog Domains
A system diagram is shown in Figure 1. The transfer function of the ASIC is labeled A.

Figure 1. Conversion Between Analog and Digital Domains, When A = 1.0
The definition of 0 dBFS as a full-scale sine wave is used by several audio analyzers, and the rms and peak values in the digital domain for a sine wave are equal for these analyzers. The same analyzers show the rms level that is measured at the output in the digital domain as being 3 dB too high compared to the definition of rms level in the analog domain. Thus, a square wave whose top and bottom are at the maximum digital codes has an rms value of 1.414 FFS or 3.01 dBFS, and the peak value is 1.0 FFS or 0.0 dBFS, whereas in the analog domain, the rms value for the corresponding square wave is 0 dBV both for peak and rms value.
For the ASIC, there are two ways of expressing the transfer function, A: one in terms of peak and one in terms of rms. This is needed because there is a 3 dB difference for the transfer function, A, which is due to an inconsistency of the peak/rms definition in the analog/ digital domain when using the commercially available digital audio analyzers, as previously explained.
Start with the general formula for transformation between the analog and digital domains, assuming the decimator (which follows the preamplifier output) has 0 dB gain in the pass band.

where A is constant in the band of interest (the audio band) and can be estimated as follows:
In rms,
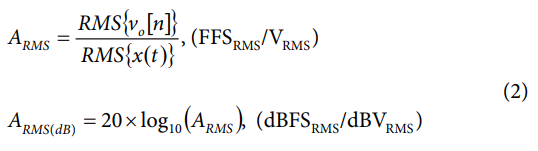
In peak,
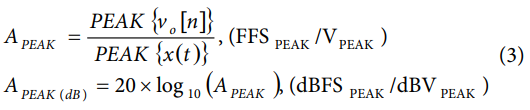
For example, if a sine wave of 14.1 mVPEAK is applied at the input to the ASIC and the output is measured as −22.1 dBFSRMS (which is the same as −22.1 dBFSPEAK), then the transfer function of the ASIC is calculated as follows:
In rms,
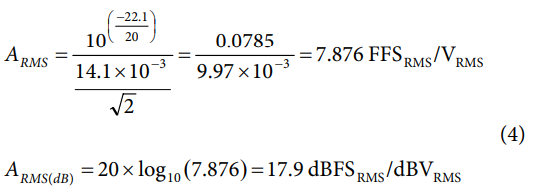
In peak,
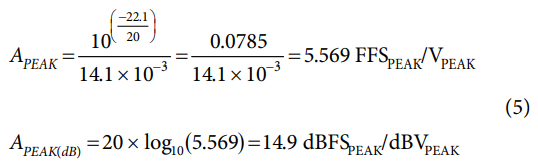
Note that peak gain, APEAK, is 3 dB lower than the rms gain, ARMS. Again, this is because the rms and peak value of the digital sine output are equal.
Many times, it is interesting to calculate the maximum input amplitude in volts of the ASIC to generate a full-scale output. Let the amplitude of x(t) be represented as VAPEAK and the amplitude of vo[n] be VDPEAK.
To achieve a maximum full-scale output (without clipping), which corresponds to an amplitude of 1.0 FFS for vo[n], the calculation for the input in the analog domain is as follows:

Measuring Noise on Microphone Preamplifier ASIC
Another measurement that is frequently done is the noise level of the ASIC (embedded inside the microphone module). The noise of the microphone preamplifier ASIC is measured digitally by decimating the output of the ASIC and running the decimated output into an audio analyzer, keeping the input to the amplifier at 0 V. However, not only is the noise at the output of interest, but also the noise referred back to the input, taking into account the transfer function of the ASIC. Assume that the gain in the pass band of the decimator is 0 dB. If the A-weighted noise is measured as −87.5 dBFSRMS at the output of the ASIC, the input-referred noise can be calculated as follows (using the same transfer function calculated previously):

Thus, the noise as seen at the input of the amplifier is 5.37 μVRMS.
Summary
Measurement devices have different methods of interpreting full scale, which affects the values recorded in testing analog and digital circuits. In some cases, the digital and analog definitions of rms differ by approximately 3 dB.
It is important to be aware of the definitions and units used in measurement devices when calculating signal levels in the analog and digital domains, and verify that these definitions are in agreement with values reported in data sheets for ASIC specifications.
Knowledge of these values allows simple calculation of parameters such as transfer function and noise.
