AN-2579: 反転昇降圧コンバータ・トポロジの設計

はじめに

反転昇降圧トポロジは正電圧入力を負出力に変換するために有用です。コンパクトなソリューションが求められる場合に、Ćuk トポロジの代替となります。反転昇降圧トポロジでは、入力電圧を入力より低い電圧（降圧モード）と高い電圧（昇圧モード）のいずれにも変換できます。しかし、Ćuk トポロジと異なり、反転昇降圧コンバータでは出力にノイズが多く発生します。そのため、電磁気的効果が大きくなります。反転昇降圧コンバータは、降圧コンバータから出力とグラウンドのリファレンスを入れ替えて作られることがよくあります。このため、入力を反転できる唯一のトポロジであるかのように「反転」と称されることも、入力を反転し負電圧に降圧できる唯一のトポロジであるかのように「反転降圧」と称されることもよくありますが、いずれも適切ではありません。実際には、反転昇降圧トポロジは、Ćuk トポロジと同様、反転した上で電圧を降圧することも昇圧することも可能です。反転昇降圧コンバータは、ダイオードを使用すれば非同期動作し、ダイオードを金属酸化膜半導体電界効果トランジスタ（MOSFET）に置き換えれば同期動作し、効率が高くなります。反転昇降圧コンバータ・トポロジは最大150W までのアプリケーションに使用されるのが一般的です。

動作

反転昇降圧コンバータの動作を図1 と図2 に示しています。

オン期間の状態では、MOSFET がオン、ダイオードはオフになります。インダクタは入力から通電されます。

オフ期間の状態では、MOSFET はオフ、ダイオードがオンになります。インダクタは出力の負荷に向けてエネルギーを放出します。

図1 と図2 の動作ではダイオードを使用しており、回路は非同期です。このダイオードをMOSFET で置き換えると、回路は同期になります。

設計アプローチ

反転昇降圧コンバータを正しく設計するためには、入力電圧の両方の極限、つまり高圧（入力電圧が最も高い）と低圧（入力電圧が最も低い）の動作を考慮することが重要です。インダクタンスと出力容量はいくつかのパラメータの関数です。このアプリケーション・ノートでは、目的のアプリケーションの仕様を満足する最小のインダクタンスと最小の出力容量が得られる条件を説明します。シーケンスに従った手順を取ります。このため、入力電圧範囲全体にわたる設計によって、正常動作に必要な全ての重要なパラメータを考慮に入れることが可能です。

設計仕様

反転昇降圧コンバータの仕様は、以下のとおりです。

V_IN(MAX) = 72 V

V_IN(MIN) = 36 V

V_OUT = −48 V

I_OUT = 2 A

ƒ_S = 350 kHz

ΔI_{OUT_TRA}(25%) = 500 mA

ΔV_{OUT_SS}(1%) = 480 mV

Δ_{VOUT_TRA}(1%) = 480 mV

設計の目的

このアプリケーション・ノートの目的は、設計サイクル全体についての手引を示すことです。例として、通信プラットフォームのような特定のアプリケーションを想定して設計仕様のセクションに列挙した目標値を検討します。通信プラットフォームでは−48V の出力は珍しくありません。高電圧、高出力、高効率のソリューションが必要だと想定しています。反転昇降圧コンバータの出力は−48V なので、入力電圧が+72V～+48V のときにはコンバータは降圧モードで動作し、入力電圧が+36V～+48Vのときには昇圧モードとなります。降圧モードと昇圧モードの両方について記載し、わかりやすいよう意図的に繰り返して説明します。数字は丸めている場合があります。

降圧モードの設計手順

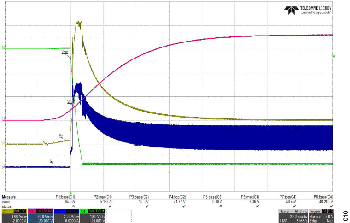
この例ではLTC3896 制御IC を使用し、出力電圧が入力電圧より低い降圧モードでの連続導通モード（CCM）動作を示します。図3 はその回路を示し、図4 は最高入力電圧における起動を示します。

出力電力は次式で計算されます。

インダクタを流れる電流は、上側MOSFET を流れる入力電流と下側MOSFET を流れる出力電流の合計です。出力電流で定格を定める降圧トポロジと異なり、反転昇降圧トポロジは、非同期コンバータではMOSFET、同期コンバータでは上側MOSFET を流れるピーク電流により定格を定めます。

平均入力電流は上側MOSFET を流れる平均電流です。

ここでη は降圧モードにおける想定効率です。

オン期間状態に上側MOSFET を流れる平均電流は、インダクタを流れる平均電流およびオフ期間状態に下側MOSFET を流れる平均電流と等しくなります。

インダクタに流れる最大電流リップルは次式で計算されます。

ここで、0.55 はインダクタの電流リップルが平均電流の55%に相当することを示しています。この電流振幅はインダクタを流れる平均電流の関数で、アプリケーションの電流レベルとインダクタの飽和電流に応じて変動しますが、一般的には40%～80%の範囲になります。

回路中で使用している上側MOSFET はInfineon Technologies のBSC520N15NS3（150V、21A）トランジスタで、最大R_DS(ON)は52mΩ です。オン期間状態には平均3.404A が流れます。したがって、デバイス全体での電圧降下は以下のように計算します。

最小デューティ・サイクルは次のようになります。

ここでV_Q2 は下側MOSFET のBSC520N15NS3 に3.404A の電流が流れるときの電圧降下です。

この期間は次のとおりです。

オン期間とオフ期間は次のように計算します。

最小インダクタンスは、最高入力電圧時で計算できます。このとき、上側MOSFET 両端の電圧が最大になり、デューティ・サイクルが最小、オン期間が最短となります。

= 44 μH → 47 μH

Würth Elektronik のインダクタ7443634700（47μH、12.2mΩ）を選択しています。

負荷抵抗は次式で計算します。

最高入力電圧時の定常状態の最小出力容量は次のように計算します。

しかし、出力の負荷電流の急な増減を補償するために、容量の追加が必要です。

この反転昇降圧コンバータには右半面ゼロ（RHPZ）という固有の条件があります。これは伝達関数の一部であり、コンバータの帯域幅を制限します。周波数が低くなるのは最低入力電圧かつ最大負荷の場合で、デューティ・サイクルが最大になり負荷抵抗が最小となります。一方、最高入力電圧に対してコンバータが降圧モードで動作するとき（デューティ・サイクルが最小）、RHPZ は高い周波数に移動し、次式に示す位置になります。

反転昇降圧コンバータの安定性を確保するため、帯域幅をRHPZ の位置の25%～33%に抑えることを推奨します。

25%の負荷過渡応答と1%のオーバーシュート／アンダーシュートを前提とすると、最小出力容量は次式で計算されます。

TDKのC5750X7S2A106K230KB（10μF、100V、2220）セラミック・コンデンサは、48V DC では4.415μF にディレーティングしますが、これを出力と並列に8 個使用して、インピーダンスを低減します。出力は−48V であるので、高耐圧のコンデンサを選択する必要があります。更に、このコンデンサはバイアス条件下で十分な容量を示す必要があります。そのため、比較的大きなセラミック・コンデンサを8 個使用します（2220）。ここでの選択は、ソリューション・サイズと出力リップル電圧のトレードオフになります。電解コンデンサとセラミック・コンデンサなど、ハイブリッド・ソリューションも選択可能で、電解コンデンサ1 個をセラミック・コンデンサ1 個と組み合わせて使用します。しかし、電解コンデンサは等価直列抵抗（ESR）と等価直列インダクタンス（ESL）が比較的高く、ハイブリッド・オプションでは定常状態のスイッチング周波数での出力リップル電圧が大きくなります。

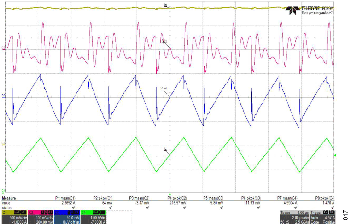
インダクタを通る最大リップル電流は、図5 に示すようになり、次式で計算されます。

インダクタを流れる電流は、上側MOSFET を流れる入力電流と下側MOSFET を流れる出力電流の合計です。

上側MOSFET、インダクタ、下側MOSFET を流れるピーク電流は、次式で計算されます。

上側MOSFET を流れる電流は台形の波形で、その実効値は次式で計算されます。

下側MOSFET の実効値電流は、式20 でD_MIN を1 − D_MIN に置き換えて同様に計算できます。

実効値電流は、入力のDC およびAC 電流とデューティ・サイクルの積の二乗平均平方根です。

出力リップル電圧は次のように2 つの項の組み合わせです。

第1項は出力コンデンサの容量性成分によるものです。

第2項は出力コンデンサの抵抗性成分、すなわちESR によるものです。

ここで358μΩ は出力コンデンサのスイッチング周波数における合成ESR です。

これらの項を足し合わせると、図6 に示すような総合出力リップル電圧が得られます。

第1項と第2項のどちらが支配的になる場合もあります。

ΔV_CがΔ_VESR よりも支配的であれば、出力は三角波のようになります。

Δ_VESR がΔV_C よりも支配的であれば、出力は台形波のようになります。

この例では、ΔV_C がΔ_VESR より大きくなっています。そのため、出力は三角波になります。

コンデンサを流れる出力リップル電流は台形波で、実効値は次式のようになります。

そのため、合成実効値電流定格値が最小でも1.638A になるよう、8 個のコンデンサを選択します。

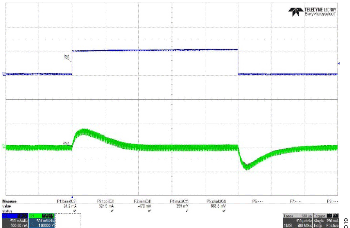
最高入力電圧におけるオーバーシュート／アンダーシュートは、図7 に示すようになり、次式で推計されます。

昇圧モードの設計手順

この例ではLTC3896 制御IC を使用し、出力電圧が入力電圧より高い昇圧モードでのCCM 動作を示します。図8 はその回路を示し、図9 は最低入力電圧における起動を示します。

出力電力は次式で計算されます。

インダクタを流れる電流は、上側MOSFET を流れる入力電流と下側MOSFET を流れる出力電流の合計です。

平均入力電流は上側MOSFET を流れる平均電流です。

ここでη は昇圧モードにおける想定効率です。

オン期間状態に上側MOSFET を流れる平均電流は、インダクタを流れる平均電流およびオフ期間状態に下側MOSFET を流れる平均電流と等しくなります。

インダクタに流れる最大電流リップルは次式で計算されます。

回路中で使用している上側MOSFET はInfineon Technologies のBSC520N15NS3（150V、21A）トランジスタで、最大R_DS(ON)は52mΩ です。オン期間には平均4.807A が流れます。したがって、デバイス全体での電圧降下は以下のようになります。

最大デューティ・サイクルは次のようになります。

ここでV_Q2 は下側MOSFET のBSC520N15NS3 に4.807A の電流が流れるときの電圧降下です。

この期間は次のとおりです。

オン期間とオフ期間は次のようになります。

最小インダクタンスは、最低入力電圧時で計算できます。このとき、上側MOSFET 両端の電圧が最小になり、デューティ・サイクルが最大、オン期間が最長となります。

= 22.2 μH → 47 μH

最高入力電圧時のインダクタンスの要件を満たすため、Würth Elektronik のインダクタ7443634700（47μH、12.2mΩ）を使用します。

負荷抵抗は次式のとおりです。

最低入力電圧時の定常状態の最小出力容量は次のようになります。

しかし、出力の負荷電流の急な増減を補償するために、容量の追加が必要です。

この反転昇降圧コンバータにはRHPZ という条件があります。これは伝達関数の一部であり、コンバータの帯域幅を制限します。周波数が低くなるのは最低入力電圧かつ最大負荷の場合で、デューティ・サイクルが最大になり負荷抵抗が最小となります。

反転昇降圧コンバータの安定性を確保するため、帯域幅をRHPZ の位置の25%～33%に抑えることを推奨します。

25%の負荷過渡応答と1%のオーバーシュート／アンダーシュートを前提とすると、最小出力容量は次式で得られます。

TDKのC5750X7S2A106K230KB（10μF、100V、2220）セラミック・コンデンサは、48V では4.415μF にディレーティングしますが、これを8 個出力に使用します。

最低入力電圧条件を満足するように、35.32μF の実効容量を選択しました。

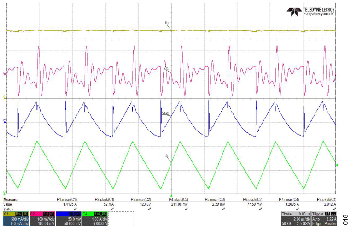
インダクタを流れる最小リップル電流は、図10 に示すようになり、次式で計算されます。

インダクタを流れる電流は、上側MOSFET を流れる入力電流と下側MOSFET を流れる出力電流の合計です。

上側MOSFET、インダクタ、下側MOSFET を流れるピーク電流は、次式で得られます。

上側MOSFET を流れる電流は台形の波形で、その実効値は次式で得られます。

下側MOSFET のRMS 電流は、式46 でD_MAX を1 − D_MAX に置き換えて同様に計算できます。

実効値電流は、入力のDC およびAC 電流とデューティ・サイクルの積の二乗平均平方根です。

出力リップル電圧は次のように2 つの項の組み合わせです。

第1項は出力コンデンサの容量性成分によるものです。

第2項は出力コンデンサの抵抗性成分によるものです。

ここで358μΩ は出力コンデンサのスイッチング周波数における合成ESR です。

これらの項を足し合わせると、図11 に示すような総合出力リップル電圧が得られます。

第1項と第2項のどちらが支配的になる場合もあります。

ΔV_CがΔV_ESR よりも支配的であれば、出力は三角波のようになります。

ΔV_ESR がΔV_C よりも支配的であれば、出力は台形波のようになります。

この例では、ΔV_C がΔV_ESR より大きくなっています。そのため、出力は三角波になります。

コンデンサを流れる出力リップル電流は台形波で、実効値は次式のようになります。

そのため、合成RMS電流定格値が最小でも2.323Aになるよう、8 個のコンデンサを選択します。

最低入力電圧におけるオーバーシュート／アンダーシュートは、図12 に示すようになり、次式で推計されます。

LTpowerCAD

反転昇降圧構成で使用するIC のLTpowerCADモデルが利用可能であれば、設計者はこのソフトウェアを使用して、設計仕様のセクションに記載の要件を満たすように、設計を作成して回路を調整することができます。適切な動的負荷レギュレーション応答を実現するように補償回路に使用する抵抗とコンデンサの組み合わせを、必要なクロスオーバに基づいてソフトウェアが提案します。しかし、LTpowerCAD モデルが利用できない場合もあります。このアプリケーション・ノートの執筆時点では、LTC3896 はLTpowerCAD でサポートされています。図13 と図14 は、このアプリケーション・ノートで説明したLTC3896 の設計の例を示しています。

LTspice

アナログ・デバイセズのスイッチング・モード電源（SMPS）のほとんどについてLTspice モデルが用意されており、時間ドメイン、周波数ドメインの最初の近似値を得るために有用です。LTspice で直接コンバータを設定しようとすると、時間のかかるシミュレーションを繰り返し行うことになります。LTspice シミュレーションではコンバータの帯域幅の概略が得られます。補償回路に使用する抵抗とコンデンサの組み合わせについて、特定の入出力条件に対してパラメータ・スイープすることで、ループを更に調整できます。

補償

内部補償がない限り、多くの反転昇降圧回路には外部補償が必要です。これは、オペレーショナル・トランスコンダクタンス・アンプ（OTA）、電流モード制御（CMC）、タイプII 補償を含む補償回路で行います。ソフトウェアによる場合でも計算による場合でも、補償の最終目標は入力電圧範囲全体にわたって十分な安定性が得られるゼロを作るような抵抗とコンデンサのペアを選択することです。

LTpowerCADもLTspice も使用できない場合には、計算に頼らざるをえません。

補償に関して推奨されるアプローチは、最低入力電圧で生ずるRHPZ の最も低い位置を計算することです。

次に、RHPZ の1/4 付近にクロスオーバを仮定します。

そして、想定のクロスオーバ周波数の10%～30%にゼロを配置します。

最後に、R_CとC_Cの値を選択してエラー・アンプにゼロを追加します。

必ず必要というわけではありませんが、出力コンデンサが作るゼロのRHPZ をキャンセルするため、もしくはノイズ抑制のために、極を配置する場合もあります。

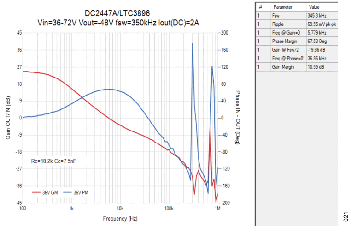
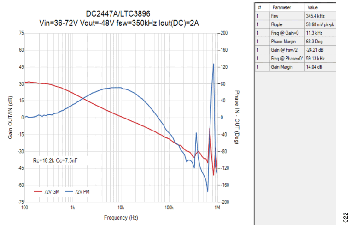
最終的なゼロの配置は、帯域幅が最も狭くなる最低入力電圧で行います。ボーデ線図によって、R_CとC_Cの組み合わせが最適かどうかがわかります。ベンチでLTC3896 をテストした結果、最適なRC は18.2kΩ となり、C_C は7.5nF のままでした。したがって、1.166kHz のゼロは初期評価の帯域幅（6.4kHz）の18%付近、実帯域幅（5.779kHz）の20%付近になります。

注記

以下に示すのは、反転昇降圧回路を設計するにあたって設計者が考慮すべき所見です。

• インダクタンスは最高入力電圧時、出力の容量は最低入力電圧時について計算します。
• インダクタを流れる最大リップル電流は最高入力電圧時に、出力の最大リップル電圧は最低入力電圧時に生じます。
• 回路の設計は入力電圧範囲の両端で行います。電流リップルと電圧リップルの要件を満たすために、出力に47μH のインダクタと有効容量35.32μF のコンデンサを使用します。
• 上側MOSFET のスイッチングに対する補償のため、正側入力と負側出力の間に小容量の高周波コンデンサを追加するのが一般的です。
• 非同期の設計であれば、下側MOSFET 両端の電圧降下V_Q2は指定の平均電流に対するダイオード両端の等価電圧降下VDに置き換えることができます。

テスト・データ

このセクションでは、最低入力電圧時と最高入力電圧時におけるLTC3896 の反転昇降圧回路のベンチでの測定結果を示します。プリント回路基板（PCB）にはR7、D1、R8 は追加されていません。

考慮すべき事項

反転昇降圧コンバータの設計にあたっては、以下の事項を考慮する必要があります。

• 適切な部品選択。入出力コンデンサの電圧定格は、それぞれに適用されるバイアスを適切に補償できるものであることが必要です。MOSFET の電圧定格は最小でもV_IN(MAX) + |V_OUT|が必要です。非同期の設計では、逆方向モードでの動作のため、ダイオードの電流定格は最小でI_SW(MAX)、ダイオードの電圧定格は最小でV_IN(MAX) + |V_OUT|が必要です。インダクタは飽和しないように選択し、ピーク・インダクタ電流より高い電流定格が必要です。
• イネーブルとレベル・シフト。反転昇降圧コンバータが常時イネーブルされている状態でない限り、イネーブル・ピンのレベル・シフトが必要で、それに対応した回路を追加します。様々な方法がありますが、PNP 型のバイポーラ・トランジスタ（BJT）もしくはP チャンネルのMOSFET（PMOS）を1つと抵抗3 つによる簡単な回路でこの機能を実現できます。LTC3896 IC のように、内部的にレベル・シフトが行われる場合もあります。
• 入力容量。コンバータの入力の実効値電流と上側MOSFETに流れる実効値電流は同じ波形になります。また振幅も同等です。上側MOSFET について計算した実効値電流値は、部品への過度なストレスを避けるように入力コンデンサの適切な組み合わせを選択する上で役立ちます。最大実効値電流時のLTspice シミュレーションによって、各コンデンサに流れる実実効値電流を、それぞれのインピーダンスの関数として算出できます。
• スイッチング周波数。最適なスイッチング周波数を選択することによって、伝導損失とスイッチング損失の間で適切なバランスを取り、インダクタンスの大小と出力容量の大小の間のトレードオフを取ることができます。
• 補償。RHPZ によってコンバータの帯域幅が制限され、昇圧モードの最低入力電圧時についての調整が必要です。LTpowerCAD でコンバータのモデルが利用可能であれば、この調整プロセスを早く進められます。利用可能でない場合には、補償回路のゼロと極を計算によって配置し、負荷レギュレーションをLTspice で検証します。出力コンデンサが全てセラミック・タイプである場合には、出力コンデンサとそのESR によって生成されるゼロをキャンセルするための高周波コンデンサの追加は必要ありません。
• ループ解析。位相マージン（PM）とゲイン・マージン（GM）を入力電圧の上限、公称値、下限にわたって検証し、約60°のPM と、スイッチング周波数の1/2 の点と位相が0°を交差する周波数の両方で少なくとも−6dB のGM を確保します（GM の値が満たされない場合、LTpowerCAD ではLTC3896の補償を行う3 番目のタブで、それぞれ−6dB と−8dB を確保しようとします）。降圧コンバータについても、図25 に示すとおり10Ω～49.9Ω の抵抗を使用して、ボード線図を作成します。ここで、反転昇降圧コンバータについては、出力が負電圧になるため、IC の帰還系へのAC 印加はR2 の下（0V近辺）に配置した抵抗の両端に対して行う必要があります。周波数応答アナライザの入力プローブのグラウンドは、入力電源とシステム・グラウンドに対してフローティングにする必要があります。このフローティングの要件を満たすためには、チータ・プラグでも十分です。しかしこの場合には、出力が−48Vであり、周波数応答アナライザの入力は通常約±15V を超える電圧に耐えられないため、損傷を与えないために10:1 の減衰が必要です。このため、チータ・プラグを避け、減衰のある高電圧差動プローブを使用します。

• 正入力と負出力の間のコンデンサ。入力と出力の間に1 つまたは複数のコンデンサを配置して、上側MOSFET の高周波スイッチングを補償する場合があります。一般的には小さい容量です。動的な負荷レギュレーションを行うためにこの容量を増加させる場合があり、出力の負荷が突然変化する場合の出力電圧変位（アンダーシュート／オーバーシュート）の低減につながります。この容量は出力容量に対して少量であることが必要です。コンデンサの電圧定格はV_IN(MAX) + |V_OUT|より大きいことが必要で、ディレーティングの考慮が、高電圧のアプリケーションでは特に必要です。
• 出力容量。算術的には出力容量を増すと出力リップル電圧が減少しますが、同時にループ帯域幅も狭くなります。そのため、出力レールに過大な容量を与えるのは逆効果になりうるため、推奨されません。計算で精密な結果を得るためには、DC バイアスを考慮する必要があります。更に精密な結果のためには、ACバイアスを導入します（ACバイアスは、出力安定化電圧に加算される出力リップル電圧）。DC バイアスとAC バイアスは重ねて加算されます。
• 負荷レギュレーション。急な負荷が変化したときに出力を安定化させる反転昇降圧コンバータの能力は、回路の出力にMOSFET を追加することでテストできます。ドレインをシステム・グラウンドに接続し、ソースを負電圧出力に接続し、入力電源およびシステム・グラウンドに対してフローティングさせたファンクション・ジェネレータでゲートをパルス駆動します。このフローティングの要件を満たすためには、チータ・プラグでも十分です。
• フィルタ処理。出力でのスイッチングから生じる電磁気的作用を抑制するために、追加の出力フィルタ処理が必要になる場合があります。この電磁気的作用は、必然的に存在するESL と結合し、特に電解コンデンサを使用し出力に高い電圧が求められる場合には、大きくなります。LC フィルタを追加すると、スイッチ動作の安定性と動作に影響します。3 端子コンデンサは効果的で有用な選択肢です。スイッチング周波数でのインピーダンスが低く、ノイズが抑制され、レイアウトのサイズも小さくできます。しかし、電圧定格が比較的低いため、電圧が比較的高いアプリケーションには適していません。最小出力インピーダンスに一致するようにスイッチング周波数を調整すると、出力リップル電圧が最適なソリューションが得られます。
• 出力電圧のクランプ。必要に応じて、出力をショットキー・ダイオードでクランプし、起動時に負電圧出力がグラウンドに対して過度に正方向に変動するのを防止します。このダイオードは、アノードを負側、カソードをグラウンド側にして両ノード間に配置します。
• 電力供給制限。コンバータは最高入力電圧で最大電力を供給しますが、場合によっては負荷電流を抑制する必要があります。これはMOSFET の損傷につながるような過度なピーク電流がスイッチング・ノードに生じるのを防止するためで、特にモノリシック・ソリューションで考慮が必要です。LTC3896 などのコントローラでは、IC に対して外付けのMOSFET を選択できる柔軟性があるので、このピーク電流による制約をあまり受けません。
• プローブ。スイッチング・ノードや出力レールの信号のプロービングには、適切な手段を用いることが重要です。ツイスト・ワイヤー・ペアを図26 に示すTektronix 131-0258-00 などのプローブ・コネクタで終端し、負電圧プローブと合わせます。この組み合わせで、トランジェントが生じた場合の電圧スパイクやリンギングの増加につながる寄生インダクタンスを低減できます。またコンデンサ等の部品にかかる機械的ストレスも抑制できます。図27 に示すのは、スペースが許せば検討可能な代替案で、SubMiniature Version A（SMA）コネクタやU.FL コネクタを直接オシロスコープの入力に接続できるBayonet Neill-Concelman（BNC）コネクタと合わせます。

• レイアウト。降圧コンバータを搭載したPCB には、構成を変更して反転昇降圧コンバータとして機能させることができるものがあります。しかし、降圧コンバータの出力をグランド・プレーンに、グランド・プレーンを負出力に変更すると、ベンチで異常で不規則な挙動を示すことがあり、混乱を招き時間の浪費につながるので望ましくありません。降圧コンバータが付いたPCB を改造するのでなく、反転昇降圧コンバータ専用のレイアウトを作成するのが最善のアプローチです。これは最高性能と低ノイズ動作を得るためのアプローチです。浮遊容量や浮遊インダクタンスを低減するために、部品の配置にも考慮が必要です。適切なレイアウトの重要性を過小評価してはいけません。図28 に標準的なDC2447A デモ・ボードのレイアウトを示します。

まとめ

反転昇降圧トポロジは負電圧のレールを作成するために有用です。

面倒に見えるかもしれませんが、このアプリケーション・ノートに示した手法と考慮事項に沿えば、回路を作成してソフトウェアでのシミュレーションとベンチでのテストができます。LTpowerCADやLTspice のようなソフトウェア・ツールによって、反転昇降圧コンバータの設計と調整ができますが、純粋な計算によって解を導くことも可能です。

参考資料

Basso, Christophe. Switch-Mode Power Supplies. The McGraw-Hill Companies, Inc., 2008.

Kessler, Matthew、アプリケーション・ノートAN-1083：スイッチング・レギュレータADP2300 とADP2301 を使った反転降圧ブーストのデザインアナログ・デバイセズ、2010

Yang, Ricky. AN-1168 Application Note. Designing an Inverting Power Supply Using the ADP2384/ADP2386 Synchronous StepDown DC-to-DC Regulators. Analog Devices, 2010.

Young, Sam. Signal Level Shifting with μModule Regulator for Positive-to-Negative Inverting Applications. Analog Devices, 2015.

著者

Enrico Reineri

Enrico Reineri received his B.S.C.E. from California State University Northridge in 2005 and his M.S.E.E. from San José State University in 2010. He worked for International Rectifier, Texas Instruments and Juniper Networks. Enrico joined Analog Devices in August 2018 and since then he has worked on a variety of topologies of DC/DC converters. He is currently a senior applications engineer with the High Performance Power group in San José, CA, U.S.A.